This article is written by Vladimir Azbel, independent consultant on tantalum capacitors, Israel, who introduces his virtual simulation tools and control algorithms for calculating the risk of anode overheating in the manufacture of tantalum capacitor.
One of the key tasks during tantalum capacitor manufacturing is the problem of acceptability of tantalum powder to ensure its electrical properties. This is due to the fact that the manufacturer of tantalum capacitors cannot formulate to the powder manufacturer what kind of powder structure he needs.
Introduction
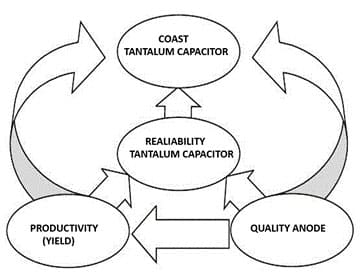
As described in my previous post on DCL leakage current optimization the anode is the basis of a tantalum capacitor (TC). Both the reliability of the TC and its cost depends on the quality of the anode, see Fig. 1.
The development of a new design of a tantalum capacitor begins with the choice of digital values for the parameters of the anode production process, see Fig. 2, which are determined by the developer and must ensure the electrical characteristics of the TC.
The technological parameters of the anode, such as choice of powder, compaction density, sintering temperature/time, electrolyte type, temperature, conductivity, and formation current, in its recipe (see Fig. 2) are the subjective choice of the developer, based on his own experience.
The technological parameters used directly affect the structure of the created composite material, which, under certain test conditions, exhibits properties characteristic of the anode, such as the anode Capacitance (CAP) and Direct current leakage (DCL).
Tantalum Powder Anode Necks
The complexity of choosing the value of each anode parameter is due to its relationship with the residual. This leads to the fact that a change in one of the technological parameters of the anode, leading to an improvement in some of its characteristics, can lead to a deterioration in others.
For example, an increase in the sintering temperature leads to a decrease in the surface area (SSA) of the powder, and the size of the inner neck between the particles increases (see Fig.3), which appears in a conflict between the capacity and the Formation Voltage (FV). see Fig 4. There must be an optimal neck size for any given FV. In its turn, CAP(CV/g) depends on FV as well.
Unfortunately, surface area and intra-particle neck size oppose each other. Figure 3 shows a powder sintered at different temperatures and the average neck size, as measured by SEM, as a function of surface area.
Powder Necks Modelling and Simulation
The purpose of the work is to make a virtual program that allows computing by way, by calculation, the numerical values of the technological parameters of the production of the anode, allowing you to provide the optimal combination of neck size and porosity of the sintered pellet, to provide the formation voltage and capacitance, for a certain anode design and predict the risk of development of aging processes in it, which are the cause of degradation of the DCL during the operation of the TC, which is not possible to do with the existing methods for calculating the anode.
The program allows the developer, by varying the various parameters of the anode, to choose the optimal values of the technological parameters of the production of the anode, according to which it is recommended to produce an experiment anode.
A virtual calculation program is considered a software tool that allows users to perform calculations or simulations in digital form.
One of the reasons for TC failures during its operation is associated with the degradation of its DCL. It is believed that the development of the latter is responsible for the aging processes in the anode, which are caused by residual stresses due to defects formed during the production of the anode The most possible reason for the formation of defects in the anode is caused by overheating of the anode, as a result of a disorder of the heat balance during the formation process, caused by an exothermic reaction, during the formation of amorphous Ta2O5
The program allows you to calculate how the structure of the anode, the critical place of which considers the neck, formed according to the technological parameters chosen by the developer, affects the heat transfer processes at the production of the anode.
The program should help the developer to choose the technological parameters for the production of the anode, allowing to achieve the heat balance in the neck area, while providing an acceptable CAP and FV.
The neck has the smallest core cross-sectional area Ta in a sintered pellet, which decreases during formation (Fig.5 ), at the similar formation current, leading to the risk of thermal imbalance and the risk of anode overheating, which causes defects in the completed anode and can be the cause of degradation of the DCL during the working of the TC.
The presented neck model makes it possible to visualize what structural components are considered in the anode neck and analyze their effect on heat transfer processes, depending on the technological parameters of the anode.
Each anode design will have its concern values of technological parameters for the production of the anode and, accordingly, its size of the Ta core and amorphous Ta2O5 in the anode neck. The program allows you to calculate the heat transfer process, that caused the creation of the anode neck.
Consider the operation of the program using the example in the Table 1. below.
Horizontally, in the table, the electrical and technological parameters of the anode are entered, chosen by the developer, and marked in blue, and the program calculates, how changes in, the size of the structural components of the anode neck during the formation process, at the same current, affect the heat transfer processes. In the program, the indicator of heat exchange in the production of the anode is the coefficient Δ. The higher the Δ value, the lower the risk of overheating. The program will allow you to calculate the size of the pore and its throat, which allows you to assess the risk of cathode impregnation during the production of TC.
And by the vertical, shown the ability to analyze, independently, the influence of each of the parameters on the change in Δ.
Because the anode is produced for a concrete design of the TC, this gives the developer information about its CAP, Rating Voltage (RV), and maximum allowable dimensions. Formation voltage FV=k*RV, where k depends on the requirements for a concrete design of the TC and usually varies from 2 to 5. The weight of a pressed pellet, for given dimensions, will depend on the pressing density determined by the developer.
The following assumptions and models were used in the program calculations:
- the shape of the particles was assumed to be spherical.
- Pore diameter of a loose powder is inversely proportional to the surface area of the particle, that is in close contact.
- Density of loose powder ˂ 3KCV/g, before pressing, was taken as 6g/cc, for powders ˃10KCV/g – 4.5g/cc.
- the decrease in pore size in the pellet, with an increase in its compression density, was calculated from the difference between the press density of the pellet, before and after pressing.
- the average particle size of the powder, during the production of a sintered pellet, remains constant and does not depend on changes in the press density, temperatures, and sintering time. Their influence is reflected in the sizes of the pore and necks. As can be seen from the proposed model (see Fig.6), this leads to a decrease in the pore size and an increase in the neck size.
- decrease in the pore size during sintering, of closely connected powder particles (see Fig.7), we describe the parameter y=√D*t, where y is taken as the difference between the centres of powder particles before (L) and after mutual diffusion (Z), atoms Ta (D inter-diffusion coefficient, t-sintering time) (see Fig.7)
- the size of the neck formed in the sintered pellet is calculated from the geometric model of the sintering of two particles (see Fig.8). The size of the neck is compared with the size of the diameter of the contact area between two sintered powder particles during their diffusion sintering (see Fig. 8), where r – is the radius of the particle; 2x – is the radius of the contact area between the particles, after sintering and y – is the difference between the diameters of the particles and their approaching after sintering Knowing the particle size of the powder (2р) and 2y=√D*t.
- neck model
- the technological process of formation the sintered pellet, leading to the creation of the anode, affects only the heat transfer process, which is calculated using the neck model / /
- CV/g of powder was assumed to be 1.25*CV/g of anode
Based on the above assumptions and the models used, a key element of the structure, the impact on which, technological parameters of the production anode, will create an acceptable structure anode, chosen the size of the primary powder particle.
The size of the powder particles directly affects the powder surface area (see Fig. 9), and the relationship between them is described by the following expression: SSA =6/d*ρ, where d is the average size of the primary powder particle, ρ is the skeletal porosity of the sample.
The relationship between BET/SSA and CB/g for tantalum powders is shown in Fig. 10.
CV/g of the powder depends not only on the particle size of the powder but also on the temperature of its sintering, see Fig.11 and neck size see Fig.12.
Data from Cabot Supermetals and Sanford Advance Materials were used to obtain information about the relationship between the primary particle size of tantalum powder, with its CV/g, and recommended their sintering temperatures.
Data on the effect of pressing density, sintering temperature, and formation voltage, on the change in pore size and their SSA, for powders of different CV/g, are presented in detail in the works of H.C. Stark
To evaluate the criterion of the value of Δ corresponding to an acceptable anode, into the program were entered values of, technological parameters anode, and capacitors which have passed reliability tests and of commercially available. This made it possible to set the value of Δ corresponding to the acceptable values of the anode parameters and test the program.
Conclusion
I propose herein my investigation and research in calculating the risk of anode overheating during manufacturing of tantalum capacitor.
My approach includes the use of virtual simulation tools and control algorithms to develop methods that allow the powder manufacturer to optimize the production process to meet the electrical requirements of the anodes. These works can be used not only in the production of the anode but also in the production of powder and reduce cost of powder production.